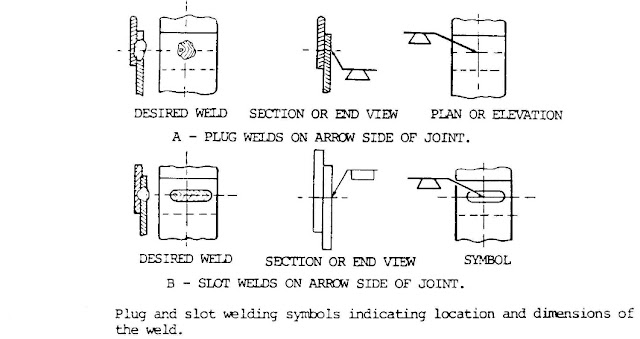
- Plug or Slot Weld BMA Engineering, Inc. Stitch or Skip Weld BMA Engineering, Inc. – 6000 9 Basic of Welding. Structural welding is a process whereby the parts to be connected are heated and fused with a molten filler.
- SLOT AND PLUG WELD CALCULATION The strength of slot and plug welds is calculated with the mean stress method as for fillet welds. In the calculation, the effective area of the slot or plug weld is taken as the area of the slot or hole.
Plug Weld Design Calculation
Other types of welds include flange welds, plug welds, slot welds, seam welds, surfacing welds, and backing welds. Joints are combined with welds to make weld joints. If you are new to MIG welding and would like simple training so you can learn quickly, without the headaches, then download my FREE beginner's guide to MIG welding. The procedure for welding size calculation for bending load is a little different than calculation of welding size for pure tension. Here we will discuss the calculation procedure called the 'line method' (BS 5950 Clause 6.8.7.2) along with examples.
Formulae_IndexWeld Time Calculator
Nomenclature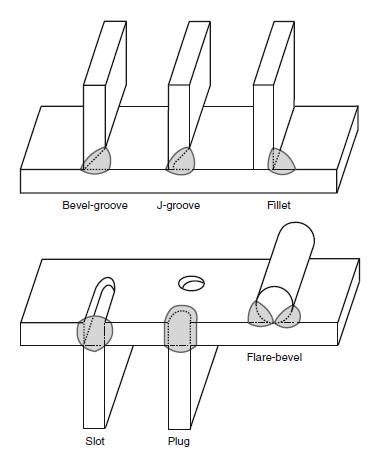

Introduction..... Relevant Standards..... Variables Associated With Welds.....
Guidance Principles..... Table Basic Weld Calcs...... Assessment of Fillet Welds.....
Examples of Fillet Welds Calcs..... Properties of Fillet Welds as lines.....
Example of torsion weld calc. using vectors..... Capacities of Fillet Welds..... Design Strength of Fillet Welds.....
| Introduction The following notes are general guidance notes showing methods ofcalculation of the strength and size of welds. Welded joints are oftencrucially important affecting the safety of the design systems. It isimportant that the notes and data below are only used for preliminary designevaluations. Final detail design should be completed in a formal wayusing appropriate codes and standards and quality reference documents Relevant Standards BS 5950-1:2000 ..Structural use of steelwork in building. Code of practice for design. Rolled and welded sections BS EN 10025-1:2004 - Hot rolled products of structural steels. General technical delivery conditions Variables related to welded joints
Guidance Principles A generous factor of safety should be used (3-5) and if fluctuatingloads are present then additional design margins should be included to allow for fatigue The table below provides provides approximate stresses in, hopefully, a convenientway. Table of bracket weld subject to direct and bending stresses
Assessment of Fillet Weld Groups ref notes and table Properties of Fillet Welds as lines Important note: The methods described below is based on the simple method of calculationof weld stress as identified in BS 5950- clause 6.7.8.2 . The other method identifed in BS 5950 - 1 clause 6.7.8.3as the direction method uses the method of resolving the forces transmittedby unit thickness welds per unit length into traverse forces (FT ) andlongitudinal forces (FL ). I have, to some extent,illustrated this method in my examples below Examples of Fillet Weld Calculations
Properties of weld groups with welds treated as lines - It is accepted that it is reasonably accurate to use properties based onunit weld thickness in calculation to determine the strength of welds as shown in the examples onthis page. The weld properties Ixx Iyy and J are assumedto be proportional to the weld thickness. The typical accuracy of this method ofcalculation is shown below... This is illustrated in the tabled values below
Note: The error identified with this method is lower as h increasesrelative to d. This error is such that the resulting designs are conservative. Example illustrating use of stress vectors
Note: The example above simply illustrates the vector method adding direct and torsional shear stresses and compares the differencein using the unit weld width method and using real weld sizes. The example calculates the stress levels in an existing weld groupit is clear that the weld is oversized for the loading scenario. The difference in the resulting values are in less than 4%. If the welds were smalleri.e 3mm then the differences would be even smaller. Table properties of a range of fillet weld groups with welds treated as lines -
Table Of Weld Capacities The fillet weld capacity tables related to the type of loading on the weld. Twotypes of loading are identified traverse loading and longitudinal loading as show below The weld loading should be such that { (FL/PL) 2 + (FT/PT) 2 } ≤ 1 The following table is in accord with data in BS 5950 part 1. Based ondesign strengths as shown in table below ... Design Strength PL = a.pw PT based on elements transmitting forces at 90o i.e θ = 45o and K = 1,25
Design Strength p w of fillet welds
|
Plug And Slot Welds
Useful Related Links
|

Introduction..... Relevant Standards..... Variables Associated With Welds.....
Guidance Principles..... Table Basic Weld Calcs...... Assessment of Fillet Welds.....
Examples of Fillet Welds Calcs..... Properties of Fillet Welds as lines.....
Example of torsion weld calc. using vectors..... Capacities of Fillet Welds..... Design Strength of Fillet Welds.....
| Introduction The following notes are general guidance notes showing methods ofcalculation of the strength and size of welds. Welded joints are oftencrucially important affecting the safety of the design systems. It isimportant that the notes and data below are only used for preliminary designevaluations. Final detail design should be completed in a formal wayusing appropriate codes and standards and quality reference documents Relevant Standards BS 5950-1:2000 ..Structural use of steelwork in building. Code of practice for design. Rolled and welded sections BS EN 10025-1:2004 - Hot rolled products of structural steels. General technical delivery conditions Variables related to welded joints
Guidance Principles A generous factor of safety should be used (3-5) and if fluctuatingloads are present then additional design margins should be included to allow for fatigue The table below provides provides approximate stresses in, hopefully, a convenientway. Table of bracket weld subject to direct and bending stresses
Assessment of Fillet Weld Groups ref notes and table Properties of Fillet Welds as lines Important note: The methods described below is based on the simple method of calculationof weld stress as identified in BS 5950- clause 6.7.8.2 . The other method identifed in BS 5950 - 1 clause 6.7.8.3as the direction method uses the method of resolving the forces transmittedby unit thickness welds per unit length into traverse forces (FT ) andlongitudinal forces (FL ). I have, to some extent,illustrated this method in my examples below Examples of Fillet Weld Calculations
Properties of weld groups with welds treated as lines - It is accepted that it is reasonably accurate to use properties based onunit weld thickness in calculation to determine the strength of welds as shown in the examples onthis page. The weld properties Ixx Iyy and J are assumedto be proportional to the weld thickness. The typical accuracy of this method ofcalculation is shown below... This is illustrated in the tabled values below
Note: The error identified with this method is lower as h increasesrelative to d. This error is such that the resulting designs are conservative. Example illustrating use of stress vectors
Note: The example above simply illustrates the vector method adding direct and torsional shear stresses and compares the differencein using the unit weld width method and using real weld sizes. The example calculates the stress levels in an existing weld groupit is clear that the weld is oversized for the loading scenario. The difference in the resulting values are in less than 4%. If the welds were smalleri.e 3mm then the differences would be even smaller. Table properties of a range of fillet weld groups with welds treated as lines -
Table Of Weld Capacities The fillet weld capacity tables related to the type of loading on the weld. Twotypes of loading are identified traverse loading and longitudinal loading as show below The weld loading should be such that { (FL/PL) 2 + (FT/PT) 2 } ≤ 1 The following table is in accord with data in BS 5950 part 1. Based ondesign strengths as shown in table below ... Design Strength PL = a.pw PT based on elements transmitting forces at 90o i.e θ = 45o and K = 1,25
Design Strength p w of fillet welds
|
Plug And Slot Welds
Useful Related Links
|